POINTS
AND LINES PROBLEMS.
"Line upon line, line upon line; here a little and there a
little."—Isa. xxviii. 10.
What are known as "Points and Lines" puzzles are found very interesting by
many people. The most familiar example, here given, to plant nine trees so that
they shall form ten straight rows with three trees in every row, is attributed
to Sir Isaac Newton, but the earliest collection of such puzzles is, I believe,
in a rare little book that I possess—published in 1821—Rational Amusement for
Winter Evenings, by John Jackson. The author gives ten examples of "Trees
planted in Rows."
These tree-planting puzzles have always been a matter of great perplexity.
They are real "puzzles," in the truest sense of the word, because nobody has yet
succeeded in finding a direct and certain way of solving them. They demand the
exercise of sagacity, ingenuity, and patience, and what we call "luck" is also
sometimes of service. Perhaps some day a genius will discover the key to the
whole mystery. Remember that the trees must be regarded as mere points, for if
we were allowed to make our trees big enough we might easily "fudge" our
diagrams and get in a few extra straight rows that were more apparent than
real.
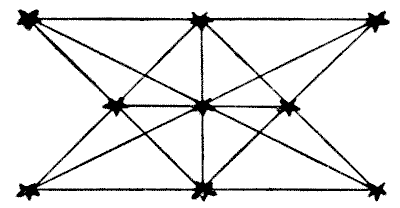
206.—THE
KING AND THE CASTLES.
There was once, in ancient times, a powerful king, who had eccentric ideas on
the subject of military architecture. He held that there was great strength and
economy in symmetrical forms, and always cited the example of the bees, who
construct their combs in perfect hexagonal cells, to prove that he had nature to
support him. He resolved to build ten new castles in his country all to be
connected by fortified walls, which should form five lines with four castles in
every line. The royal architect presented his preliminary plan in the form I
have shown. But the monarch pointed out that every castle could be approached
from the outside, and commanded that the plan should be so modified that as many
castles as possible should be free from attack from the outside, and could only
be reached by crossing the fortified walls. The architect replied that he
thought it impossible so to arrange them that even one castle, which the king
proposed to use as a royal residence, could be so protected, but his majesty
soon enlightened him by pointing out how it might be done. How would you have
built the ten castles and fortifications so as best to fulfil the king's
requirements? Remember that they must form five straight lines with four castles
in every line.
Solution 207.—CHERRIES
AND PLUMS.
The illustration is a plan of a cottage as it stands surrounded by an orchard
of fifty-five trees. Ten of these trees are cherries, ten are plums, and the
remainder apples. The cherries are so planted as to form five straight lines,
with four cherry trees in every line. The plum trees are also planted so as to form five straight
lines with four plum trees in every line. The puzzle is to show which are the
ten cherry trees and which are the ten plums. In order that the cherries and
plums should have the most favourable aspect, as few as possible (under the
conditions) are planted on the north and east sides of the orchard. Of course in
picking out a group of ten trees (cherry or plum, as the case may be) you ignore
all intervening trees. That is to say, four trees may be in a straight line
irrespective of other trees (or the house) being in between. After the last
puzzle this will be quite easy.
Solution 208.—A
PLANTATION PUZZLE.
A man had a square plantation of forty-nine trees, but, as will be seen by
the omissions in the illustration, four trees were blown down and removed. He
now wants to cut down all the remainder except ten trees, which are to be so
left that they shall form five straight rows with four trees in every row. Which
are the ten trees that he must leave?
Solution 209.—THE
TWENTY-ONE TREES.
A gentleman wished to plant twenty-one trees in his park so that they should
form twelve straight rows with five trees in every row. Could you have supplied
him with a pretty symmetrical arrangement that would satisfy these
conditions?
Solution 210.—THE
TEN COINS.
Place ten pennies on a large sheet of paper or cardboard, as shown in the
diagram, five on each edge. Now remove four of the coins, without disturbing the
others, and replace them on the paper so that the ten shall form five straight
lines with four coins in every line. This in itself is not difficult, but you
should try to discover in how many different ways the puzzle may be solved,
assuming that in every case the two rows at starting are exactly the same.
Solution 211.—THE
TWELVE MINCE-PIES.
It will be seen in our illustration how twelve mince-pies may be placed on
the table so as to form six straight rows with four pies in every row. The
puzzle is to remove only four of them to new positions so that there shall be
seven straight rows with four in every row. Which four would you remove,
and where would you replace them?
212.—THE
BURMESE PLANTATION.
A short time ago I received an interesting communication from the British
chaplain at Meiktila, Upper Burma, in which my correspondent informed me that he
had found some amusement on board ship on his way out in trying to solve this
little poser.
If he has a plantation of forty-nine trees, planted in the form of a square
as shown in the accompanying illustration, he wishes to know how he may cut down
twenty-seven of the trees so that the twenty-two left standing shall form as
many rows as possible with four trees in every row.
Of course there may not be more than four trees in any row.
Solution 213.—TURKS
AND RUSSIANS.
This puzzle is on the lines of the Afridi problem published by me in
Tit-Bits some years ago.
On an open level tract of country a party of Russian infantry, no two of whom
were stationed at the same spot, were suddenly surprised by thirty-two Turks,
who opened fire on the Russians from all directions. Each of the Turks
simultaneously fired a bullet, and each bullet passed immediately over the heads
of three Russian soldiers. As each of these bullets when fired killed a
different man, the puzzle is to discover what is the smallest possible number of
soldiers of which the Russian party could have consisted and what were the
casualties on each side.
Solution
Previous Next
|